Fractals with Octave: Original Function Studied by Gaston Julia

The story so far

• Classic Mandelbrot and Julia
• More on the Classic Sets
• Other Polynomials
• Trigonometric and Exponential Functions
• Burning Ship

After another long pause, here is the sixth instalment of this series on fractals with Octave. In this article, we have a look at the complex series that started is all, the one that Gaston Julia was interested in.

Once Upon A Time: The Original Fractal Function

The classic Mandelbrot set we all know and love is derived from quite a simple series, described in the very first article of this series. Gaston Julia was interested in a slightly more complex series, as explained by Paul Bourke:

z_n+1=z⁴ + z³/(z-1) + z²/(z³+4z²+5) + c

The initial condition is:

z₀=0

The Mandelbrot Set

We can produce a Mandelbrot set of that equation with the mandelbrot function that we created in previous articles.

octave:1> Mj=mandelbrot(-1.4+1.05i,1.4-1.05i,320,64,
> @(z,c) z.^4.+z.^3./(z-1)+z.^2./(z.^3.+4*z.^2.+5).+c;
octave:2> imagesc(Mj)

And here is the result:

Original Julia Series, Mandelbrot Set

We can then zoom on the bottom right corner of that image.

octave:1> Mjz=mandelbrot(0.25-0.65i,0.45-0.8i,320,64,
> @(z,c) z.^4.+z.^3./(z-1)+z.^2./(z.^3.+4*z.^2.+5).+c;
octave:2> imagesc(Mjz)

Original Julia Series, Mandelbrot Set x10

And again.

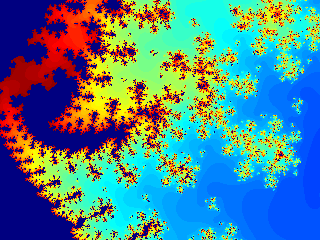
octave:1> Mjzz=mandelbrot(0.378-0.725i,0.398-0.74i,320,64,
> @(z,c) z.^4.+z.^3./(z-1)+z.^2./(z.^3.+4*z.^2.+5).+c;
octave:2> imagesc(Mjzz)

Original Julia Series, Mandelbrot Set x100

And again!

octave:1> Mjzzz=mandelbrot(0.3863-0.7314i,0.3883-0.7329i,320,64,
> @(z,c) z.^4.+z.^3./(z-1)+z.^2./(z.^3.+4*z.^2.+5).+c;
octave:2> imagesc(Mjzzz)

Original Julia Series, Mandelbrot Set x1000

The Julia Set

Now, let's have a look at the Julia set for the same series and for c=0.3873-0.7314i, which is the point towards which we zoomed on in the Mandelbrot set.

octave:1> Jj=julia(-1.2+1.6i,1.2-1.6i,240,64,0.3873-0.7314i,
> @(z,c) z.^4.+z.^3./(z-1)+z.^2./(z.^3.+4*z.^2.+5).+c;
octave:2> imagesc(Jj)

Original Julia Set, c=0.3873-0.7314i

Let's zoom into that picture.

octave:1> Jjz=julia(0.2+0.1i,0.4-0.05i,320,64,0.3873-0.7314i,
> @(z,c) z.^4.+z.^3./(z-1)+z.^2./(z.^3.+4*z.^2.+5).+c;
octave:2> imagesc(Jjz)

Original Julia Set, c=0.3873-0.7314i, x10

And again.

octave:1> Jjzz=julia(0.366+0.09i,0.386+0.075i,320,64,0.3873-0.7314i,
> @(z,c) z.^4.+z.^3./(z-1)+z.^2./(z.^3.+4*z.^2.+5).+c;
octave:2> imagesc(Jjzz)

Original Julia Set, c=0.3873-0.7314i, x100

And again!

octave:1> Jjzzz=julia(0.366+0.09i,0.386+0.075i,320,64,0.3873-0.7314i,
> @(z,c) z.^4.+z.^3./(z-1)+z.^2./(z.^3.+4*z.^2.+5).+c;
octave:2> imagesc(Jjzzz)

Original Julia Set, c=0.3873-0.7314i, x1000

The End

This is the last article in this series. I hope you've enjoyed it, even if it took me a very long time to finish it. Have a play with the Octave functions described throughout the articles, modify them, improve them, there's a lot of fun stuff to do and amazing pictures to create with fractals.